How Unschooling Kids Learn: Environment as Classroom: Part 1 of the soldering series

Lots of people ask how unschoolers learn without classrooms or teachers. The perhaps paradoxical answer is that they have classrooms and teachers, just not in the traditional sense. The kids' (7 y.o. No. 1, 5 y.o. No. 2, and 3 y.o. No. 3), teachers are whoever they happen to be around when they're curious about something. Their classrooms tend to be anywhere but our house, where we all typically decompress for a few hours around dinnertime before we head off to bed. We do have haunts form time to time where we spend more time learning. When the kids were younger, we had a favorite coffee shop with a grass lawn and an upstairs. We'd hang out upstairs practicing numbers and division.

Since we've come to San Francisco, our favorite learning haunt has changed. Google came the closest to what we use for a classroom when they implemented rolling study halls:

More times that not, the gang and I find ourselves discussing 'school' subjects on San Francisco public transit. Which isn't to say that One, Two, and Three don't do science at playgrounds, art at museums, and socialize all over town. Transit just happens to be where we usually are when I can work with the kids.

Here's an example. Two weeks ago, the gang and I visited a hackerspace here in San Francisco. We'd already been playing with plug-in breadboarding for LED and logic circuits, so One was intrigued when she saw what looked like a breadboard, but not quite. Asking our host about it, she found out that it was a breadboard for soldering. She immediately said she wanted to learn to solder!

For those not in the know, soldering is used to permanently attach wires and components to circuit boards. So, instead of a circuit with connections that looks like this pluggable breadboard:

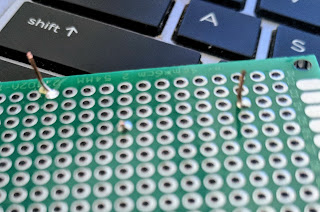
You wind up with connections that look like the ones shown in more detail here.

Those are the same size wires permanently soldered to metal pads. The whole affair is pulled off by heating the pad and wire till they're hot enough to melt the solder. If done correctly, the soldering iron, (the heat source), never touches the solder, just the wire and the pad.

As we trundled along to the hackerspace in our rolling study hall, (better known as the M train), I found myself explaining the same thing to One that I just detailed above. One and I both learn really well verbally, so I'd already started talking about how to solder, as I looked around looking for something I could use as an example wire. Then I noticed it was right in front of me, staring out of the train wall. The square peg in a round hole used to lock the wall panel in place suddenly looked like nothing more to me than a wire centered inside a solder pad.

Using my ballpoint pen as the pretend soldering iron, I showed One how she was going to solder, being careful to heat the pad and wire from one side while feeding the solder from the other.

With this model for an example, One nailed actual soldering at the hackerspace. Those are her first solder joints pictured above! Of course, there was more to it, like stripping insulation off wires, but I'll uncover that next time, (silly pun totally intended).

So there you go, our unschooling classrooms tend to move these days. Even when they don't they're usually somewhere outside the house. Let's face it, that's where the rest of the world is happening!

It's always cool to hear how other homeschooling families are doing things. What's your favorite place for learning?

The Valentine's Day Magnetic Monopole

There's an assymetry to the form of the two Maxwell's equations shown in picture 1. While the divergence of the electric field is proportional to the electric charge density at a given point, the divergence of the magnetic field is equal to zero. This is typically explained in the following way. While we know that electrons, the fundamental electric charge carriers exist, evidence seems to indicate that magnetic monopoles, the particles that would carry magnetic 'charge', either don't exist, or, the energies required to create them are so high that they are exceedingly rare. That doesn't stop us from looking for them though! Keeping with the theme of Fairbank[1] and his academic progeny over the semester break, today's post is about the discovery of a magnetic monopole candidate event by one of the Fairbank's graduate students, Blas Cabrera[2]. Cabrera was utilizing a loop type of magnetic monopole detector. Its operation is in concept very sim

Cool Math Tricks: Deriving the Divergence, (Del or Nabla) into New (Cylindrical) Coordinate Systems

Now available as a Kindle ebook for 99 cents ! Get a spiffy ebook, and fund more physics The following is a pretty lengthy procedure, but converting the divergence, (nabla, del) operator between coordinate systems comes up pretty often. While there are tables for converting between common coordinate systems , there seem to be fewer explanations of the procedure for deriving the conversion, so here goes! What do we actually want? To convert the Cartesian nabla to the nabla for another coordinate system, say… cylindrical coordinates. What we’ll need: 1. The Cartesian Nabla: 2. A set of equations relating the Cartesian coordinates to cylindrical coordinates: 3. A set of equations relating the Cartesian basis vectors to the basis vectors of the new coordinate system: How to do it: Use the chain rule for differentiation to convert the derivatives with respect to the Cartesian variables to derivatives with respect to the cylindrical variables. The chain

More Cowbell! Record Production using Google Forms and Charts

First, the what : This article shows how to embed a new Google Form into any web page. To demonstrate ths, a chart and form that allow blog readers to control the recording levels of each instrument in Blue Oyster Cult's "(Don't Fear) The Reaper" is used. HTML code from the Google version of the form included on this page is shown and the parts that need to be modified are highlighted. Next, the why : Google recently released an e-mail form feature that allows users of Google Documents to create an e-mail a form that automatically places each user's input into an associated spreadsheet. As it turns out, with a little bit of work, the forms that are created by Google Docs can be embedded into any web page. Now, The Goods: Click on the instrument you want turned up, click the submit button and then refresh the page. Through the magic of Google Forms as soon as you click on submit and refresh this web page, the data chart will update immediately. Turn up the:

Copasetic Flow

Search This Blog